Principles of thermography

Here we describe the principles of thermography as implemented by modern thermography cameras.

The discovery of infrared radiation

In 1800, the German Friedrich Wilhelm Herschel, better known as the English king's astronomer, George III, and discoverer of the planet Uranus, was looking for a way to protect his eyes when observing the Sun through telescopes and, when testing colored glass samples, observed that some let more heat pass through than others. With the aid of a prism and three mercury thermometers with the bulbs painted black, Hershel measured the temperature of the various color components of sunlight refracted through the prism and incident on a screen.. Noticed an increase in temperature from violet to red, as previously observed by Landriani, However, also observed that the highest temperature peak occurred in the dark region, beyond the red. With this he concluded that there was, in that region, invisible light heating thermometers. The region was named Thermometric Spectrum and radiation the name of Black Heat. Decades later, this region of the electromagnetic spectrum came to be called Infrared Region and radiation, of Infrared Radiation. Herschel published the results of his experiments in a paper for Philosophical Transactions of Royal Society.

Principles of thermography – Figure 1 – The experience of Sir William Herschel

Below you can see a video about this discovery.

Principles of thermography – Heat and temperature

Heat is the transfer of energy from one region to another as a result of a temperature difference between them. This energy originates from the agitation of the molecules of which matter is made and its transfer occurs from the hottest region to the coldest region.. The heat is, therefore, a transitory phenomenon, which ceases when there is no longer a temperature difference.

Principles of thermography – Figure 2 – Heat and temperature

If two objects are in thermal equilibrium with a third object, so they are in thermal equilibrium with each other. As a result of this, there is a certain state attribute or property that describes the thermodynamic states of objects that are in thermal equilibrium with each other, and this is called temperature.

Principles of thermography – Heat transfer modes

There are three heat transfer modes:

• Driving,
• Convection
• Radiation

All heat transfer processes occur through one or more of these three modes. Infrared thermography is based on the measurement of heat flow by radiation and is, therefore, closely related to the mode of heat transfer by radiation.

Principles of thermography – Figure 3 – Heat transmission processes

Principles of thermography -Conduction

Conduction can be defined as the process by which energy is transferred from a region of high temperature to a region of lower temperature within a medium. (solid, liquid or gaseous) or between different media in direct contact. This mechanism can be viewed as the transfer of energy from more energetic particles to less energetic particles of a substance due to interactions between them.. The heat source directly excites the particles, which transfer part of their energy to neighboring particles and these in turn transfer it to other particles.. The flow intensity depends on the thermal conductivity of the material, with metals having high thermal conductivity and insulators having low thermal conductivity.

The amount of heat per unit time through a flat plate is represented by the figure and expressed by Fourier's law in Eq..

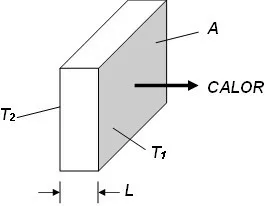
Principles of thermography – Figure 4 – Flat plate with the arrow indicating the direction of heat flow.

In which,

 Q/Dt [J.s^-1] is the heat flow by conduction.

 k [J.s^-1.m^-1.K^-1] is the thermal conductivity coefficient of the material.

 A [m²] is the area of ​​the section through which heat flows by conduction, measured perpendicular to the flow direction;

T2–T1 [K] is the temperature gradient in the section.

L [m] is the section thickness.

The proportionality factor k (thermal conductivity) which arises from the Fourier equation is a property of each material and expresses the greater or lesser ease that a material presents to heat conduction. The numerical values ​​of k vary over a wide range depending on the chemical constitution, physical state and temperature of materials. When the value of k is high, the material is considered thermal conductor and, otherwise, thermal insulator. In some materials such as aluminum and copper, k varies very little with temperature, but in others, like some steels, k varies significantly with temperature. In these cases, adopt, as an engineering solution, an average value of k over a temperature range.

The variation, for some materials, of thermal conductivity with temperature is shown in figure.

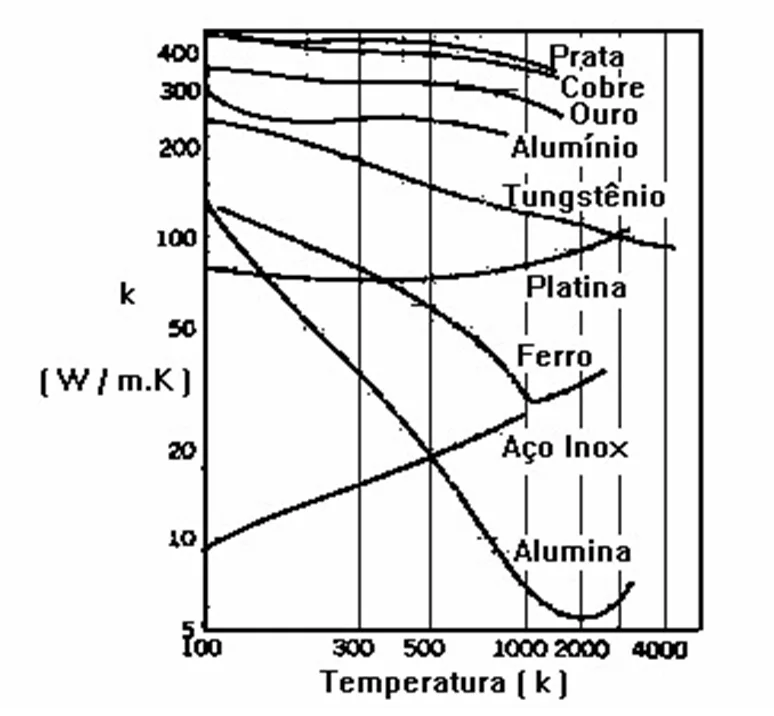
Principles of thermography – Figure 5- Variation of thermal conductivity with temperature.

The figure presents an example of heat transfer by conduction.

Principles of thermography – Figure 6 – Heat transfer by conduction from the hand to the wall in the DMC

Below you can see a video with an example of heat conduction.

Principles of thermography – Convection

Convection can be defined as the process by which energy is transferred from hot portions to cold portions of a fluid through the combined action of: heat conduction, energy storage and mixing movement.

Transformer oil and water from refrigeration systems are examples of fluids that produce convective cooling.. O ar, forced or not, Blowing equipment in a substation is another example of a fluid that can drastically affect the temperature of that equipment.

Principles of thermography – Figure 7 – Convection in a heating liquid

Heat transferred by convection, in the unit of time, between a surface and a fluid does not have a simple equation, but an approximation can be achieved through the relationship proposed by Isaac Newton:

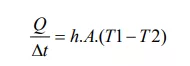
In which,

Q/Dt [J.s^-1] is the heat flow transferred by convection.

h [J.s^-1.m^-1.K^-1] is the convection heat transfer coefficient.

A [m²] is heat transfer area.

T1 – T2 [K] is the temperature difference between the surface and the fluid.

The convection heat transfer coefficient h dependent on several factors such as: the surface orientation, fluid type, fluid speed and surface type.

Principles of thermography – Radiation

Radiation can be defined as the process by which heat is transferred from a surface of high temperature to a surface of lower temperature when such surfaces are separated in space, even if there is a vacuum between them. The energy thus transferred is called thermal radiation and is in the form of electromagnetic waves that travel at the speed of light..

Heat transfer by radiation is the basis for measuring temperature through infrared thermography, which detects radiation originating from the object under inspection, more specifically infrared radiation.

Principles of thermography – Figure 8 – Heating using radiation

Principles of thermography – Infrared radiation

All objects above absolute zero (0 K or -273.16° C) emit thermal radiation due to the thermal agitation of atoms and molecules of which they are made. The greater this agitation, the hotter the object is and the more radiation it emits.

Principles of thermography – The electromagnetic spectrum

Thermal radiation can be emitted in the ultraviolet ranges, visible, infrared and even in the microwave range of the electromagnetic spectrum. However, for typical temperatures found on Earth, most thermal radiation is emitted within the infrared range. Therefore, thermography chambers are manufactured with detectors that respond to this spectrum range.

Thermography detects the infrared radiation emitted by the inspected object, which is invisible to the human eye, and transforms it into visible thermal images, with the possibility of converting it into temperature readings.

Within the electromagnetic spectrum, infrared radiation is located between the visible radiation region and the microwave radiation region. These regions are divided arbitrarily, depending on the methods used to produce and detect radiation.

Principles of thermography – Figure 9 – Electromagnetic spectrum

Infrared radiation, as well as radiation from different regions of the electromagnetic spectrum, basically obey the same laws. Propagate in a straight line, reflect, refracted, are absorbed, interfere, feature beam scattering, can be focused and travel, in a vacuum, at a speed of approximately 3 x 10⁸ m/s.

Principles of thermography – Short wave and long wave

The infrared spectrum can further be divided into subregions.

Manufacturers of infrared thermography equipment, refer to equipment that works in the range of 8 µm a 14 µm Long Wave (Long-Wave – LW) and equipment in the range of 3 µm a 5 µm Short Wave (Short-Wave – SW).

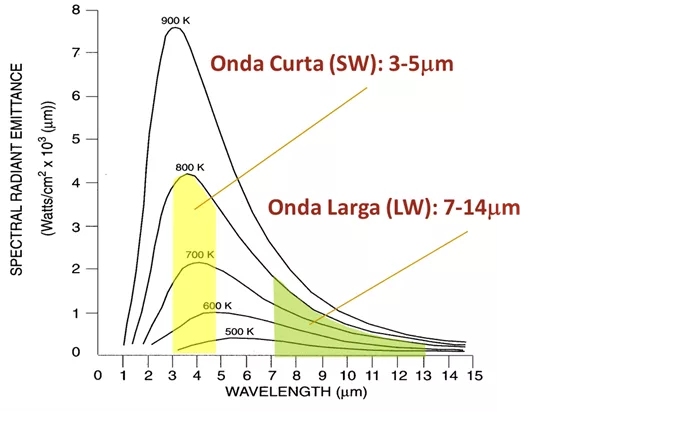
Principles of thermography – Figure 10 – Wavelengths

There is also the use of the terms, Reflected Infrared for radiation ranging from 0,75 µm a 1,2 µm and Thermal Infrared for radiation beyond 2 µm.

Principles of thermography – The black body

Gustav Robert Kirchhoff em 1860 proposed the term Black Body as a body capable of absorbing all incident radiation, independent of its wavelength, its direction of incidence and its polarization. The radiation emitted by it would have a spectral distribution dependent only on its temperature. For such a body to be in thermodynamic equilibrium, it should radiate energy at the same rate it absorbs it. Therefore, a black body, besides being a perfect absorber, It is also a perfect emitter.

Principles of thermography – Stefan-Boltzmann's Law

In 1879, Josef Stefan concluded through experimental measurements that the total amount of energy radiated by a Black Body is proportional to the fourth power of its absolute temperature., same conclusion obtained through theoretical means by Ludwig Eduard Boltzmann in 1884, resulting in Stefan-Boltzmann's law.

Principles of thermography – Figure 11 – Stefan-Boltzmann's law

Principles of thermography – Plank's law

German physicist Max Karl Ernst Ludwig Planck, in 1900, formulated a theory to explain the behavior of radiation emitted by Black Bodies.

Max Planck, by exhibiting his work before the Berlin Physics Society, presented a formula in agreement with the experimental results, introducing a hypothesis: the thermal movement of atoms and molecules, responsible for generating electromagnetic waves, can oscillate freely at any frequency, but the emission of radiation resulting from this oscillation occurs discontinuously, that is, through pulses, called quanta and the energy emitted by them is proportional to the oscillation frequency of the particles, in the form:

          E = h f⋅

In which, E [J] is the energy.

h [J.s] is Planck's constant = 6,6260755 x 10^-34 [J.s].

f [Hz] is the frequency of the radiation.

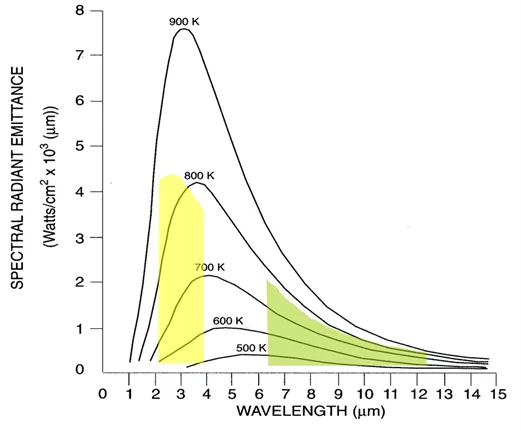
Principles of thermography – Figure 12 – Planck's Law, for blackbody radiation, expresses the spectral radiance as a function of the wavelength and temperature of the black body.

The total radiance (M_b) of a Blackbody can be obtained by integrating Planck's formula over the wavelength λ = 0 up to wavelength λ = ∞.

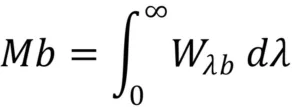
                  Resulting in:

          M_b = pT⁴ 

In which,

M_b [W.m^-2] is the total radiance.

p  [W.m^-2.K^-4] is the Stefan-Boltzmann constant = 5,67051 x 10^-8 [W.m^-2.K^-4].

T [K] is the absolute temperature.

Graphically, the total radiance (M_b) represents the area under the Planck curve for a specific temperature. The Figure shows the total radiance of the Black Body (M_b) at a given temperature (T).

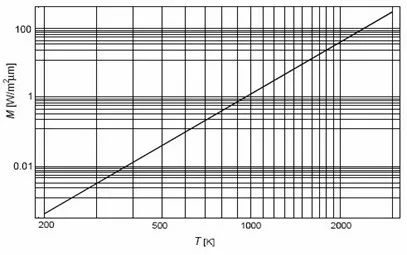
Principles of thermography – Figure 13 – Total Black Body Radiance (M_b) at a given temperature.

Principles of thermography – Radiation laws for real emitters

The radiation laws described so far refer only to the Black Body. Real-world objects do not follow these laws across much of the spectrum, although they can approach the behavior of a Blackbody in certain wavelength ranges.

The radiance of real objects is lower than that of a Black Body and it is necessary to characterize some radiative properties of such objects.

There are two relationships between the radiative properties of materials that are very important. The first is the one that relates absorbance, reflectance and transmittance in the radiative energy balance, and the second is Kirchhoff's law that relates absorption and emissivity.

A real body when hit by radiation can present the following phenomena:

• A fraction of the incident radiation can be absorbed α.
• A fraction of the incident radiation can be reflected ρ.
• A fraction of the incident radiation can be transmitted τ.

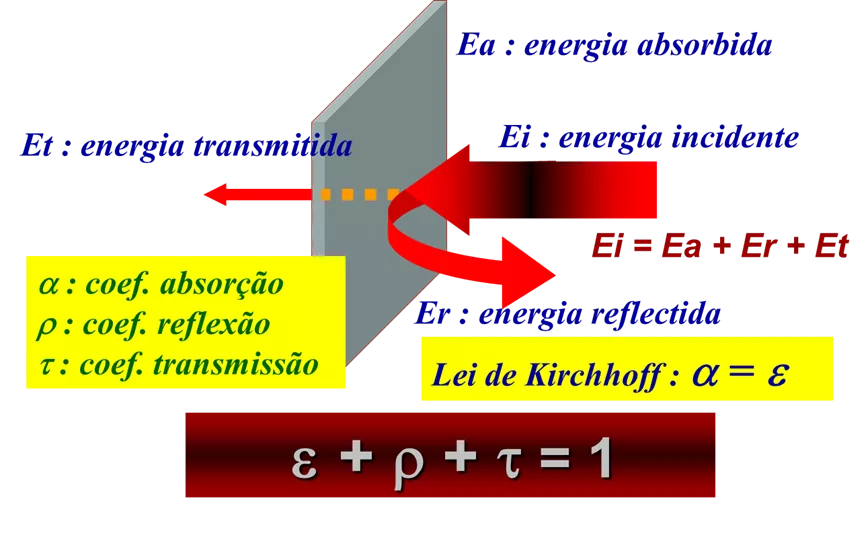
Principles of thermography – Figure 12 – Graphical representation of the radiation incident on a real object and the possible fractions of absorbed radiation (a_l), reflected (r_l) and transmitted (t_l).

The intensity of these phenomena depends on the wavelength of the incident radiation., therefore:

• The α spectral absorption_l is the ratio of the spectral energy absorbed by a body to the radiation incident on it.
• The spectral reflection ρ_l is the ratio of the spectral energy reflected by a body to the radiation incident on it.
• The spectral transmission τ_l is the ratio of the spectral energy transmitted by a body to the radiation incident on it.

The sum of the three coefficients αλ, ρλ and τλ for the same wavelength λ, results in total radiation:

al + rl + tl = 1

The figure shows the radiation incident on a real object and the possible fractions of absorbed radiation (a_l), reflected (r_l) and transmitted (t_l).

For opaque objects, t_l = 0 and the relationship simplifies to:

         al + rp = 1

Principles of thermography – The emissivity

Another property called emissivity (e) describes the fraction of radiance produced by any surface in relation to that produced by a Black Body at the same temperature. Therefore, the spectral emissivity (e_l) is the ratio of the spectral radiance of a surface to the radiance of a Blackbody at the same temperature and wavelength:

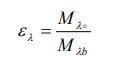
In general, There are three types of radiation sources, characterized by the way in which spectral radiance varies with wavelength:

• Black Body, for which ε_l = e = 1.
• Gray body, for which ε_l = ε = constant and less than 1.
• Selective radiator, for which ε varies with wavelength.

The spectral distribution curves and spectral emissivity of these three radiation sources are presented in the following Figures.

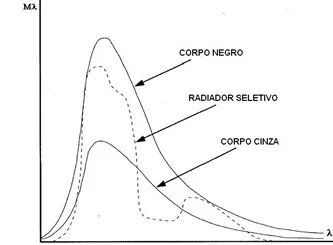
Principles of thermography – Figure 15 – Spectral radiance of the three types of radiation sources.

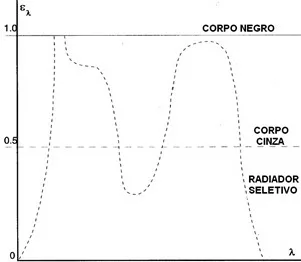
Principles of thermography – Figure 16 – Spectral emissivity of the three types of radiation sources.

According to Kirchhoff's law, the ability of a body to absorb incident energy at a given wavelength λ is equivalent to the ability of that body to emit energy at the same wavelength.

For better understanding, consider an opaque object that is in thermal equilibrium. Its temperature remains constant and, according to Kirchhoff's law, For this to happen, the emitted radiation must be balanced with the absorbed radiation.. On the other hand, If the emitted radiation is greater than the absorbed radiation, the object will cool down.. If the absorbed radiation is greater than the emitted radiation, the object will heat up.

The capacity of a surface, in emitting and absorbing radiation at a given wavelength, is related to spectral emissivity, surfaces with high emissivity have a greater capacity to emit and absorb radiation.

Principles of thermography – Factors affecting emissivity

The main factors that affect emissivity are:

• Material type,
• Surface roughness,
• Physical and chemical structure
• Material thickness.

Principles of thermography – Cavity effect

Each time radiation is reflected, its intensity I is reduced by the reflectance ρ of its surface. If the radiation is reflected N times, the resulting intensity is:

           I = I_O ⋅_r^N

In which,

I [W.s^-1] is the final intensity.

I_O [W.s^-1] is the initial intensity

N is the number of times the radiation is reflected.

Multiple reflections have a huge effect on reflected intensity. This fact, known as the “cavity effect”, works as a means of increasing the emissivity of low-emissivity surfaces as illustrated in Figure.

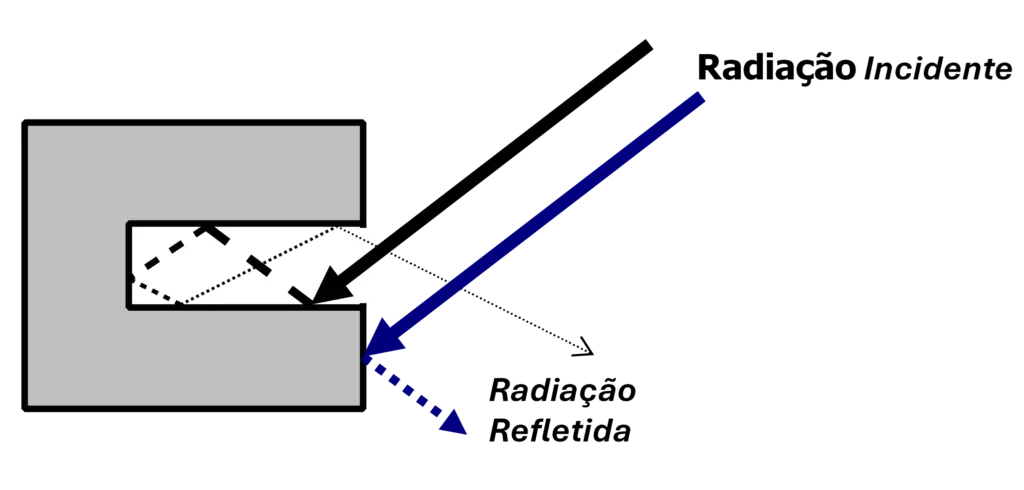
Principles of thermography – Figure 13 – Radiation falling on a low-emissivity surface.